Quotient Rule Review

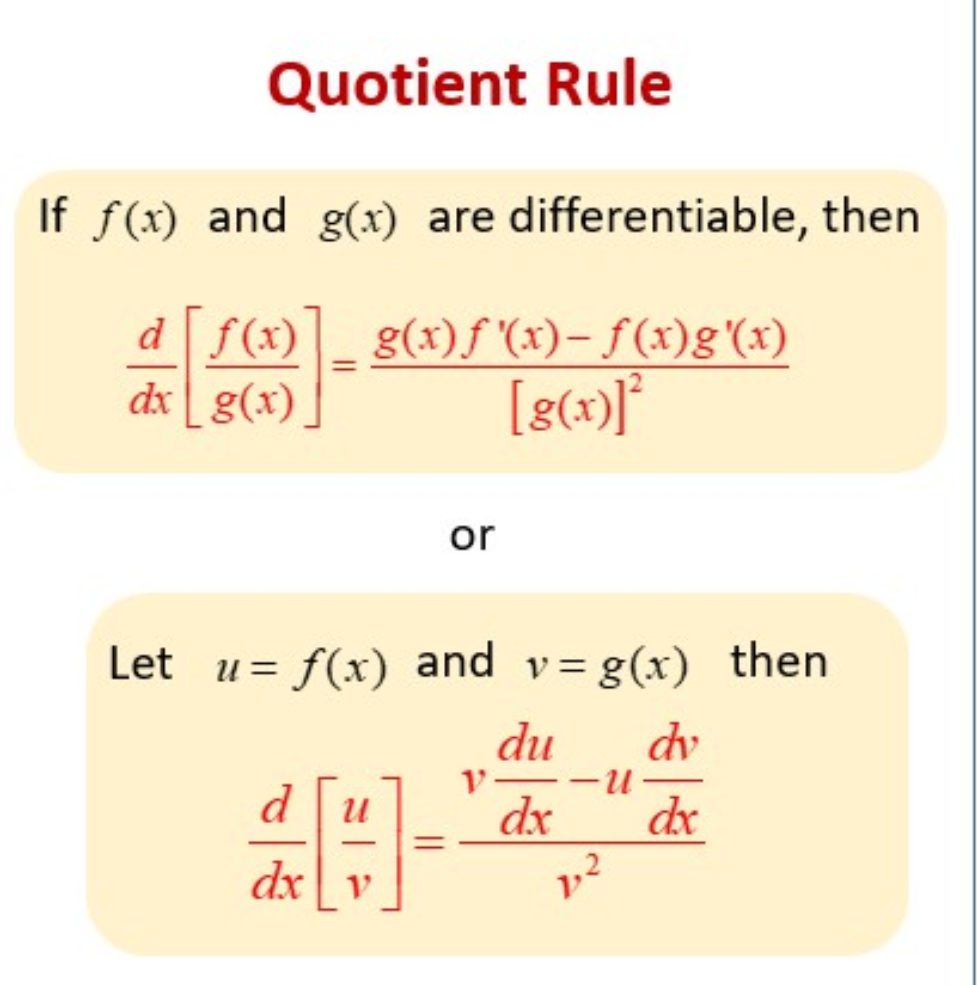
Quotient Rule Derivative: The remainder rule is a formula for picking up the derivative of a remainder of two functions. It makes it somewhat easier to keep track of all of the terms. Let’s look at the creed. In calculus, the remainder rule is a arrangement of finding the derivative of a function that is the proportion of two differentiable functions. Let where both and are differentiable.

The remainder rule is a formula for taking the subordinate of a remainder of two functions. … The formula states that to find the derivative of f(x) divided by g(x), you must: Pick up g(x) times the derivative of f(x). Then from that commodity, you must subtract the commodity of f(x) times the by-product of g(x).

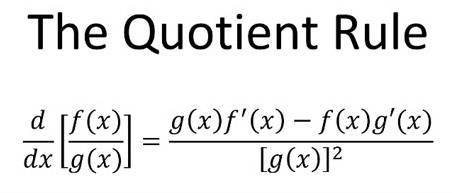
Derivative Quotient Rule

The quotient rule is a formula for finding the derivative of a fraction. This page will show you how to take the derivative using the quotient rule. Type the numerator and denominator of your problem into the boxes, then click the button.

There are rules we can follow to find many derivatives.

For example:

The slope of a constant value (like 3) is always 0
The slope of a line like 2x is 2, or 3x is 3 etc
and so on.

Here are useful rules to help you work out the derivatives of many functions (with examples below). Note: the little mark ’ means “Derivative of”, and f and g are functions.

Common Functions	Function	Derivative
Constant	c	0
Line	x	1
	ax	a
Square	x²	2x
Square Root	√x	(½)x^-½
Exponential	e^x	e^x
	a^x	ln(a) a^x
Logarithms	ln(x)	1/x
	log_a(x)	1 / (x ln(a))
Trigonometry (x is in radians)	sin(x)	cos(x)
	cos(x)	−sin(x)
	tan(x)	sec²(x)
Inverse Trigonometry	sin^-1(x)	1/√(1−x²)
	cos^-1(x)	−1/√(1−x²)
	tan^-1(x)	1/(1+x²)

Quotient Derivative Rule

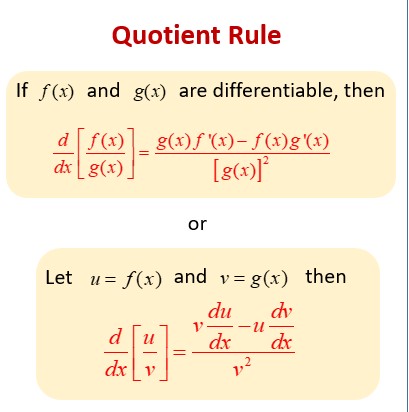
In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions.^[1]^[2]^[3] Let $f(x)=g(x)/h(x),$ where both $g$ and $h$ are differentiable and $h(x)\neq 0.$ The quotient rule states that the derivative of $f(x)$ is $f'(x) = \frac{g'(x)h(x) - g(x)h'(x)}{[h(x)]^2}.$

Derivative Product And Quotient Rule

The following problems compel the use of the remainder rule. In the following discussion and solutions the derivative of a function h(x) will be denoted by or h‘(x) . The quotient rule is a academic rule for discriminating complications where one business is divided by other. It follows from the limit interpretation of derivative and is given by

$tex2html_wrap_inline63$ .

Remember the rule in the following way. Always start with the “bottom” function and end with the “bottom” function squared. Note that the numerator of the quotient rule is identical to the ordinary product rule except that subtraction replaces addition. In the list of problems which follows, most problems are average and a few are somewhat challenging.

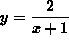- PROBLEM 1 : Differentiate $tex2html_wrap_inline65$ .
  Click HERE to see a detailed solution to problem 1.
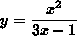- PROBLEM 2 : Differentiate $tex2html_wrap_inline67$ .
  Click HERE to see a detailed solution to problem 2.
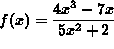- PROBLEM 3 : Differentiate $tex2html_wrap_inline69$ .
  Click HERE to see a detailed solution to problem 3.
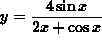- PROBLEM 4 : Differentiate $tex2html_wrap_inline71$ .
  Click HERE to see a detailed solution to problem 4.
- PROBLEM 5 : Differentiate $tex2html_wrap_inline73$ .
  Click HERE to see a detailed solution to problem 5.
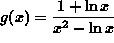- PROBLEM 6 : Differentiate $tex2html_wrap_inline75$ .
  Click HERE to see a detailed solution to problem 6.
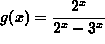- PROBLEM 7 : Differentiate $tex2html_wrap_inline77$ .
  Click HERE to see a detailed solution to problem 7.

Partial Derivative Quotient Rule

The quotient rule is a formula for taking the derivative of a quotient of two functions. It makes it somewhat easier to keep track of all of the terms. Let’s look at the formula.

If you have function f(x) in the numerator and the function g(x) in the denominator, then the derivative is found using this formula:

In this formula, the d denotes a derivative. So, df(x) means the derivative of function f and dg(x) means the derivative of function g. The formula states that to find the derivative of f(x) divided by g(x), you must:

Take g(x) times the derivative of f(x).
Then from that product, you must subtract the product of f(x) times the derivative of g(x).
Finally, you divide those terms by g(x) squared.

The quotient rule formula may be a little difficult to remember. Perhaps a little yodeling-type chant can help you. Imagine a frog yodeling, ‘LO dHI less HI dLO over LO LO.’ In this mnemonic device, LO refers to the denominator function and HI refers to the numerator function.

Let’s translate the frog’s yodel back into the formula for the quotient rule.

LO dHI means denominator times the derivative of the numerator: g(x) times df(x).

less means ‘minus’.

HI dLO means numerator times the derivative of the denominator: f(x) times dg(x).

over means ‘divide by’.

LO LO means take the denominator times itself: g(x) squared.

What is the derivative of a quotient?

QUOTIENT RULE

In words, this can be remembered as: “The derivative of a quotient equals bottom times derivative of top minus top times derivative of the bottom, divided by bottom squared.”

Is it possible to find the derivative of a quotient without using the quotient rule?

However, just because a function has a portion bar does not mean the Quotient Rule is the BEST practice to find the derivative. In this video I discuss when it is best to use the Quotient Rule and when it is not. Then, the derivative of a quotient is unearthed without use of the Remainder Rule.

What is the meaning of quotient rule?

Similar to product rule, the quotient rule is a way of differentiating the quotient, or division of functions. The quotient rule is defined as the quantity of the denominator times the derivative of the numerator minus the numerator times the derivative of the denominator all over the denominator squared.

Quotient Rule Second Derivative